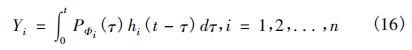FPCWAY

Analysis of Vibration Characteristics of FPCBs _2_Theory of Vibration Analysis

2.Theory of Vibration Analysis

    Modal shapes are inherent dynamic characteristics of a structure, which can describe the specific vibration conditions of the structure in a particular frequency domain, directly affecting the dynamic performance of the structure. When a structure is excited by spontaneous excitation or external excitations such as impacts, the resulting vibration is composed of modal shapes at various natural frequencies superimposed with different coefficients. When the excitation frequency is close to or equal to one of the structure's natural frequencies, the entire structure will exhibit the specific vibration form corresponding to that mode, and the vibration level under this condition will be significantly enhanced.

    The generalized vibration differential equation of a structure under external excitation can be expressed as:

    In the equation, M, C, and K represent the mass matrix, damping matrix, and stiffness matrix of the structure, respectively, and they are all positive definite symmetric matrices. U is the displacement response of the structure, and P(t) is the excitation load.

    If the structure is undamped or the damping is in the form of proportional damping or other classical damping, the resulting modal vectors are all real vectors. The vibration differential equation can be written as:

    The modal vector matrix Φ and the natural frequency matrix p of the structure are orthogonal, meaning that:

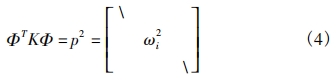
    When there is classical damping, the following relationships hold:

    The displacement vector U of the structure and the modal response vector Y are related by a transformation:

    Substituting equation (6) into equation (1), we obtain the vibration differential equation expressed in terms of the modal response vector:

    Multiplying equation (7) on the left by Φ^T and using equations (3) to (5), we get:

    Where PΦ and CΦ are defined as:

    Extracting any single equation from equation (8), we get:

    The equation is known as the dynamic equation in modal coordinates, and it can be solved using the same methods as for single-degree-of-freedom systems. From this, we can obtain the impulse response function for equation (11):

    Combining equations (12), we obtain the modal impulse response function matrix:

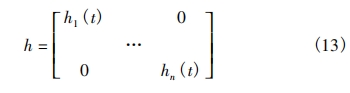
    The frequency domain response function corresponding to h_i(t) is given by:

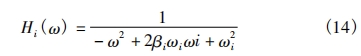
    Combining equations (14), we obtain the modal frequency domain response function matrix:

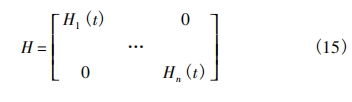
    The components of the modal response vector are obtained as follows: